The Schumann Resonance, 432Hz & Solfeggio Frequencies

by Randy Stack

Prologue: The Schumann resonances are perhaps the most powerful and all-pervasive LF EM (Low-Frequency Electro-Magnetic) phenomena to which we will ever be exposed on our humble planet Earth (not counting man-made LF EM sources like 50H/60Hz power distribution and more localised culprits like electric railways and such). The following graphic shows real-time frequency data (updated every 15 minutes) for its first four harmonic modes from the Schumann Resonance monitoring apparatus at the Space Observing System in Tomsk, Russia:

Schumann resonances as of (UTC+7) Tomsk, Tomsk Oblast, Russia
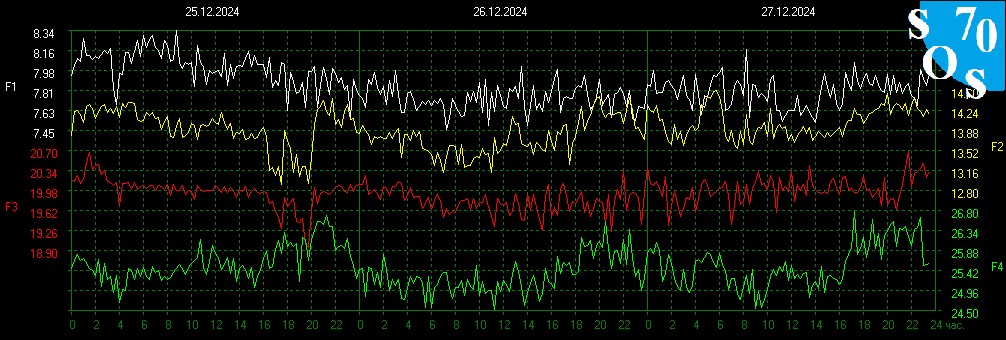(hover over image to zoom)

When I look at something scientific, I tend to have a fairly immediate and instinctual reaction; I’ll either think, “Ooh, that’s interesting!” coupled with an innate desire to explore it further or, “Pah, that’s just pure rubbish!” discounting it off-hand as being nothing more than far-fetched, whimsical, “new age” nonsense.

However, now that I’m much older and hopefully at least a little wiser, I’ve decided to begin challenging myself a bit more when I have that negative reaction to something new with which I’m presented. In other words, if I say something is rubbish, why should anyone respect my opinion? And, for that matter, why should I? Since there /may/ just be the possibility I’m being closed-minded, jaded and/or judgemental, and I just couldn’t live with myself being dogmatic and shallow like that.

So if my impulsive, intuitive mind says it’s rubbish, I don’t want to let it off the hook so easily… I want to sit my mind down across the table from me and PROVE WHY it thinks that way! Anyway, that’s my new life’s resolution, and I’m sticking to it (and in fact have been for some time now!)!

Sometimes, I prove /myself/ wrong, like when I found out I was >0.000006Hz off in my 2002 calculation of the frequency standard for scientific scale at C256.0̅Hz! I’d calculated A430.538971Hz using the 32-bit floating-point mathematics available to me at the time, whereas the far more accurate A430.5389646099018460319362438314061Hz was derived by recalculating in my new 128-bit floating-point environment.

The reason I was calculating the concert pitch reference for C256.0̅Hz was that everyone was banging on about A432 and how that was the natural A as it was meant to be from ancient times and beyond, but that seemed like rubbish to me because pitch reference is relative. The important things are temperament and intonation. But everyone was so adamant about A432, I just had to check it out!

And, when I did, the literature said one of the reasons it was so important was that middle-C became 256Hz (a natural power of 2!) and this was historically significant for some unspecified reason; whereas, at A440, middle-C was 261.63Hz (well, actually, it’s 440/2^(9/12)) or 261.6255653005986346778499935233047Hz, give or take, but I’ll allow that for rounding). However, when I applied the same rigorous proof to A432, I found that middle-C was much closer to 256.8687368405877504109799936410628Hz (or 432/(2^(9/12))) which, when doubled for the next octave, became 513.7374736811755008219599872821256Hz… nowhere even /near/ a natural power of 2! ⍣

And /that/, dear friends, is why I had to calculate my ultimate A430.5389646099018460319362438314061Hz concert pitch reference, because 430.5389646099018460319362438314061/ (2^(9/12))=256.0̅Hz! ⍢

[It might be worthwhile to note here that my calculations are based upon 12-tone equal temperament, since that is the most ubiquitous standard amongst modern musical instruments. I could just as easily have used meantone, Pythagorean, Helmholtz or just intonation. In just intonation, for example, the interval ratio from C to A is 5:3, so you’d need a concert pitch of A426.6̅ to achieve a middle-C of 256.0̅Hz (since 426.6̅/(5/3)=256.0̅), whereas A440 would give C264.0̅ and A432 yields C259.2, justly-tuned.]

But I digress…

…ANYWAYS, I’d been given some research materials by our London headquarters the other day and, whilst perusing these as fodder for a meeting the next day upon the health & well-being benefits of various psychoacoustic stimuli, I’d noticed my old acquaintance A432 cropping up amongst the topics therein! It was nestled neatly there between references to the Schumann Resonance and Solfeggio Scales, with a notation citing one would “need to understand Schumann Resonance to understand these frequencies”.

Fair enough. All of these are real things with real meanings. While I may not necessarily agree with the “mathematical justification” and conspiracy theories surrounding A432 as a tuning standard (and don’t even get me started on the latter!), it’s just as good as any; you can use A440, A432, even my A430.5389646099018460319362438314061! It’s all a matter or what sounds and works best for you (orchestras in Cuba, for example, tune to A436 – right dead centre between A432 & A440 – why? because violin strings are expensive and hard to obtain there, so tuning lower than the A440 standard makes them last longer!). A432 is, in fact, the recommended tuning for those using Pythagorean intonation, since the Pythagorean ratio from C to A is 27:16, and 432/(27/16)=256.0̅ for a true 256Hz middle-C!

When the pitch standard of the tuning fork was invented in 1711 by John Shore, trumpeter and lutenist for “Messiah” composer Georg Friederich Händel and trumpet voluntary maestro Henry Purcell, there was no agreed-upon concert pitch for A like we have today. Thus, the tuning forks of the time varied greatly; one from Händel in 1740 was pitched at A422.5, whilst a later example from 1780 was A409. A tuning fork belonging to Ludwig van Beethoven circa 1800 clocked in at A455.4! Prior to 1711, the monochord was the most scientific tuning standard, but these, too, varied greatly due to natural environmental fluctuations as well as bridge position. So, suffice it to say that tuning standards like A432, A440 and such are a relatively modern invention, not something conceived in the ancient of days. It just annoys me when people start making attributions to esoteric wisdom of the past without foundation, as it’s been my own life’s work to take /legitimate/ examples of such and adapt it for re-realisation upon modern technology.

Now, I am neither a doctor of musicology nor medieval studies (I rely on my mates Yoel & Vinny in such matters, who /are/ Ph.D.s in those fields, respectively {and special thanks to Yoel for the hours of historical music theory lessons he’d given me in my preparations for this blog!}), but I /do/ know that Solfeggio is what we were taught in kindergarten through the third grade since, in the fourth, we were taught to read music notation on manuscript paper. For those of you unfamiliar with Solfeggio by name, let me refer you to “The Sound of Music” (no, not some deep literary work of musicological significance, but the 1960s musical starring Julie Andrews). In it, she instructs the Von Trapp children in singing through the song “Do-Re-Mi”. ←/THIS/ is the Solfeggio Scale!

The information detailed in the research paper I was reading appeared to imply the Solfeggio Scale was created by medieval monks and had some direct connection to the Schumann Resonance – supposedly the “heartbeat” of our planet Earth – and A432 tuning. Well, they were right on /one/ count; it /was/ created by a medieval monk! Guido de Arezzo was his name and his musicological treatise “Micrologus” (written around 1025/6) was the definitive reference on the topic through the Middle Ages. He’d created solfège as a means of musical instruction, borrowing the solmisation syllables from the Latin hymn “Ut Queant Laxis” (ut-re-mi-fa-sol-la, corresponding to the six notes in the hexachord on which the hymn is based {is all we had back then; ti didn’t come ’til later, and do replaced ut in the 1600s). Coincidentally, Guido is /also/ regarded as the father of modern music notation and also credited with the design of the moveable bridge for the monochord! Coincidence?! ⍤

The “Solfeggio Frequencies” cited in the research paper of 396Hz, 432Hz, 528Hz, 639Hz, 741Hz & 852Hz have nothing to do with the genuine medieval hexachord nor Guido de Arezzo’s work. In fact, these frequencies don’t even fall within a single octave’s range (as was the intention of the instructional solfège)! At best, these map loosely – using the recommended A432 – to a musical scale of G, A, C, E, F# and another A (albeit a terribly sharp, inharmonious one, based upon their A432 pitch standard!), whereas the historical Solfeggio matches the C, D, E, F, G, A scale perfectly (see sheet music above), which would make the /true/ Solfeggio frequencies, based upon A432 and assuming Pythagorean tuning; 256Hz, 288Hz, 324Hz, 341⅓Hz, 384Hz & 432Hz (I’ll spare you the maths, unless you ask)! ⍩

[Well, it happened, someone asked me to, “Please show all work?” So…]

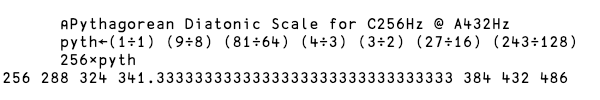
Beyond that, who knows? Perhaps the 396Hz, 432Hz, 528Hz, 639Hz, 741Hz & 852Hz frequencies, when sounded together or in different permutations to yield highly particular, complex intervallic “harmonies”, engender some other psychoacoustic or physioacoustic effect I’ve yet to see scientifically documented. We all know that a singular frequency on its own is relative and has little or no impact, unless it’s precisely resonant and phase-synchronous (or perfectly phase-/asynchronous/ {how’s that for an oxymoron?!}) with some other vibratory body, and that all of the mathematical “magic” occurs in the complex interplay /between/ multiple frequencies!

As an aside, I was just chatting with my L.A. partner Don about the arbitrary nature of frequency standards (i.e. how one doesn’t become important until everyone in the orchestra or, in a more microcosmic sense, every key on your piano is tuned relative to it). He was saying how most people say that a perfect-5th of C is G, not understanding that, without the C root, G has no interval whatsoever. As Marvin Gaye & Kim Weston so succinctly put it back in 1965, “It Take Two” (at the /minimum/) frequencies to manifest interval and harmony. However, I shall extend this definition as well to say that harmony and interval /can/ exist within a singular complex waveform as well, since these are comprised of multiple frequencies, magnitudes and phases shaping the whole (e.g. the perfect-5th of a C sawtooth wave is its 3rd harmonic {and its 6th harmonic, and its 12th, and…}). Then again, the /perfect/-5th of a C is not /always/ C-G, but that’s another topic for another blog.

But… and here’s the clincher! …the paper stated emphatically that we “need to understand Schumann resonance to understand these frequencies“! So, in keeping with my new life’s resolution of keeping an open mathematical mind, I read on…

“Solfeggio frequencies resonate in harmony
with Schumann resonance at 8 Hz“

…AAAARRRRRRGGGGGHHHH!! The Schumann Resonance is /not/ 8Hz!

What the Schumann resonances /are/ is the result of the waveguide-like behaviour of the spherical shell-shaped resonant cavity formed between the Earth’s surface and its ionosphere when stimulated by electromagnetic discharge events such as lightning strikes. Erm, just imagine a ball-shaped, planet-sized ocarina with a solid ball within its core and the space between its inner and outer balls beginning to vibrate or ring out (like a bell) with an electromagnetic standing-wave when struck by the excitational force of a sferic (radio atmospheric signal) impulse lightning breath gently blown into it! ⍨

Waveguides (like an ocarina or an organ pipe or a bottle you blow over the top of) are governed by very specific mathematics; the same maths that make up music and harmony. Now, normally, you would use the speed of sound (approximately 343m/s in air at sea-level at an ambient temperature of 20˚C) to calculate the fundamental frequency of a waveguide but, since the Schumann resonances are electromagnetic rather than acoustic wave phenomena, we must use the speed of light instead… but the rest of the rules remain the same.

An ideal electromagnetic waveguide would have a fundamental frequency of 𝑐/(2π𝑎) where 𝑐 is the speed of light and 𝑎 is the radius of the core (which is, in our case, the Earth). However, the Earth is not an ideal waveguide, as there are differences between the polar and equatorial radii due to its oblate spheroid shape. Also, despite being taught that the speed of light is constant that, too, varies depending upon the index of refraction for its conductive medium (which is why your arm looks impossibly bent if you stick it in an aquarium, for example). So, considering all these variations, I made the following calculations…

…and, taking the extents of these values (the minimum equatorial mode in air and maximal polar mode in a vacuum, i.e. the two middle calculations), I’d arrived at a fundamental frequency range between ∼7.47859Hz & ∼7.5059479Hz (and was heartened to later learn that the University of Bath and Max Planck Institute had arrived at a similar figure in their jointly-published “50 Years of Schumann Resonance” paper!). Winfried Otto Schumann, esteemed founder of his eponymous resonance, went on even further to extend the fundamental ƒ₀ calculation to V(𝜎)/(2π𝑎) as well as add a √𝑛(𝑛+1) multiplicative addendum (where sigma 𝜎 is the conductivity factor modulating the speed of light and 𝑛 is the harmonic number)  to the equation, taking into account the spherical geometry as well as a damping factor (think in terms of the damper pedal on a piano, or a cloth touched to a ringing bell) due to the finite conductivity in the upper ionospheric boundary of the waveguide, yielding…

…ANYWAYS, where were we? Ah, yes, the Schumann resonances. But, beyond being some esoteric theoretical vibratory phenomenon, the Schumann resonances are real, measurable, all-encompassing and extremely low frequency electromagnetic signals which vary from time to time due to fluctuations in the properties of their Earth / ionosphere waveguide. While the accepted ƒ₀ (or ƒ₁, if you’re an engineer!) fundamental is approximately 7.83Hz, this value modulates as much as 0.5Hz on any given day, even moreso on days with a -y with an earthquake in (or, to be fair, any seismic activity).

And, when we’re talking the sub-8Hz range, a full octave way down in those mega-bass frequency spans only occupies a total of ∼4Hz! So, just imagine with me, having to cram all 12 chromatic notes of the musical scale into a meagre 4Hz octave, and you get (leaving out all the five ♯ sharps & ♭ flats, but again using A432 and Pythagorean intonation {why buck an already-established trend?}), the notes of the Solfeggio Scale (see where I’m going with this?) would be…

…and rounding off any of the fractional frequencies above would result in a terribly inharmonic diatonic scale!

So, during the course of a single day, the Schumann Resonance can vary as much 0.5Hz, which would be as much as a whole tone off in our 4Hz-8Hz octave scale above. And, if we take the measured and agreed upon Schumann Resonance fundamental frequency of 7.83Hz and transpose that up by five octaves to get to the middle-C range, 7.83×2⁵=250.56Hz (which is a whole lot closer to middle-B♯, to my reckoning {look closely at my tuning fork photo waaay back toward the beginning of this blog}, than C256.0̅Hz)!

So, if you take nothing else away from this blog, just remember that rounding off to whole numbers can be quite dangerous and deceptive in the pursuit of scientific and mathematical truth. Why, by that reasoning, why don’t we just truncate our sublimely beautiful and natural constants π pi, 𝑒 and φ phi (the Golden Mean)? Pi can be 3, 𝑒 can be 2 and the golden mean can just be 1 (remember how, in that research paper I’d read, they’d rounded 256.8687368405877504109799936410628Hz /down/ to 256Hz? {I know, that was a long time ago} so please forgive my highly irregular and improper rounding)!

But, don’t get me wrong, I get annoyed with engineers as well when I ask them the speed of light in a vacuum and they reply, “3e8.” It’s not bloody 3e8, it’s 299,792,458m/s! But I /will/ accept 2.99792458e8, if you wish to use your fancy engineer-speak. :~)

And, to any of you who’ve actually endured reading this far, I applaud your persistence and determination, I apologise for my often / sometimes ranting, rambling manner, and I thank you…

⍨R❤️⨳

[P.S. Those of you who know me will be well aware that I have no issue with genuine magick, but pseudo-scientific clap-trap being portrayed as real science – or, worse, real magick – just gets my hackles up for its blatant (yet, perhaps, unawares or unintentional) deviousness and diabolical nature in misleading and depriving innocent and sincere seekers and followers of their truth.]

[P.P.S. And just one more post-postscript addendum for the individual who’d inquired of me, whilst debating the relative merits of alternative tuning systems, intonations and temperaments on microtonal synthesizers, “Yes, but how does that convert to MIDI?” {see ↓below↓}]